1 2 1 3 1 4

In zeta function regularization the series is replaced by the series the latter series is an example of a dirichlet series when the real part of s is greater than 1 the dirichlet series converges and its sum is the riemann zeta function ζ s on the other hand the dirichlet series diverges when the real part of s is less than or equal to 1 so in particular the.

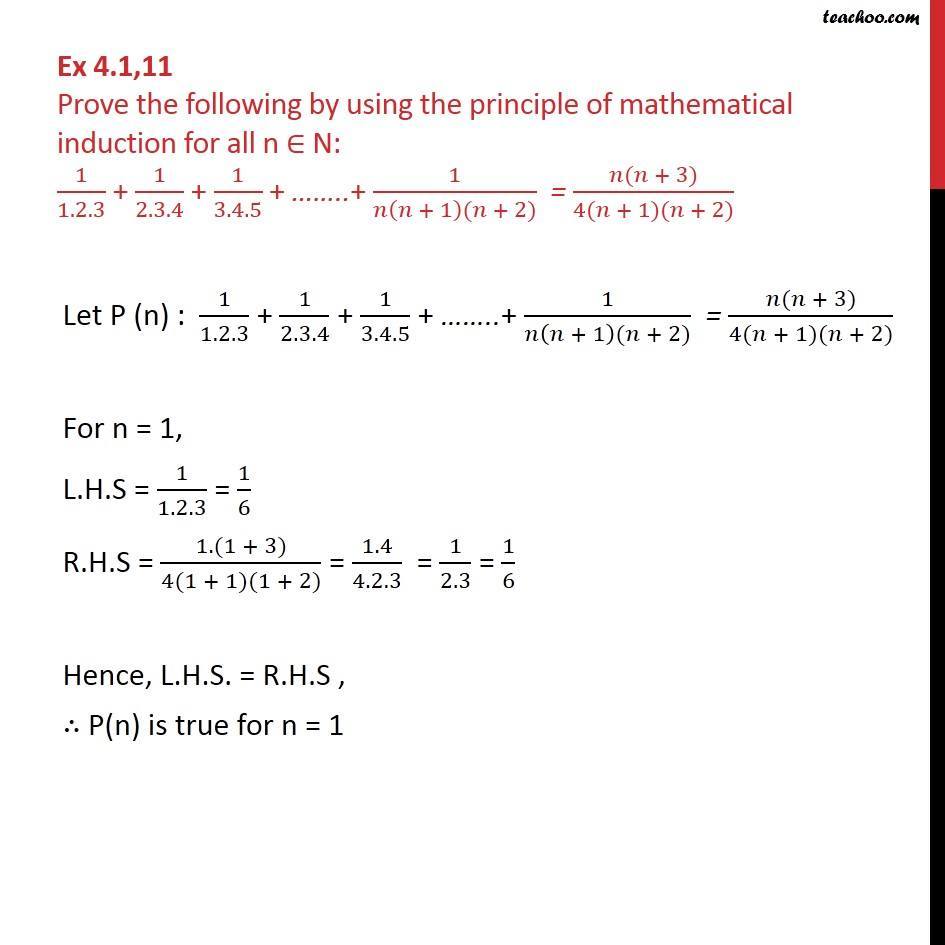
1 2 1 3 1 4. Program to find the sum of the series 1 1 4 1 9. Sum of the reciprocals sum r 1 n 1 r h n where h n is the nth harmonic number. In mathematics 1 2 3 4 is an infinite series whose terms are the successive positive integers given alternating signs using sigma summation. If inverse of a sequence follows rule of an a p i e arithmetic progression then it is said to be in harmonic progression in general the terms in a harmonic progression can be denoted as.

1 a 1 a d 1 a 2d 1 a 3d. 2 1 2 2 2 3. The series 1 4 1 16 1 64 1 256 lends itself to some particularly simple visual demonstrations because a square and a triangle both divide into four similar pieces each of which contains 1 4 the area of the original. Tomcat version 7 0 only supports j2ee 1 2 1 3 1 4 and java ee 5 and 6 web modules.

Program to find sum of series 1 1 2 1 3 1 4. Laila malik author of program to print the sum of series 1 1 2 1 3 1 4. Which then also led to. Sum of the reciprocals of the squares sum r 1 n 1 r 2 pi 2 6 sum r 1 n beta k n 1 k where beta x y is the beta function.

Related articles and code. The statement that 1 2 1 4 1 8 1 16 is absolutely convergent means that the series 1 2 1 4 1 8 1 16 is convergent. Cannot change version of project facet dynamic web module to version 3 0. 1 n last updated.

In the figure on the left if the large square is taken to have area 1 then the largest black square has area 1 2 1 2 1 4. In fact the latter series converges to 1 and it proves that one of the binary expansions of 1 is 0 111. In mathematics the infinite series 1 2 1 4 1 8 1 16 is an elementary example of a geometric series that converges absolutely. Program to determine the sum of the following harmonic series for a given value of n.

I won t go into a full explanation as it too complex.